(506) 2240-6804
3D printing mathematical sculpture
Fresh Off the 3D Printer: Henry Segerman’s Mathematical Sculptures | Science
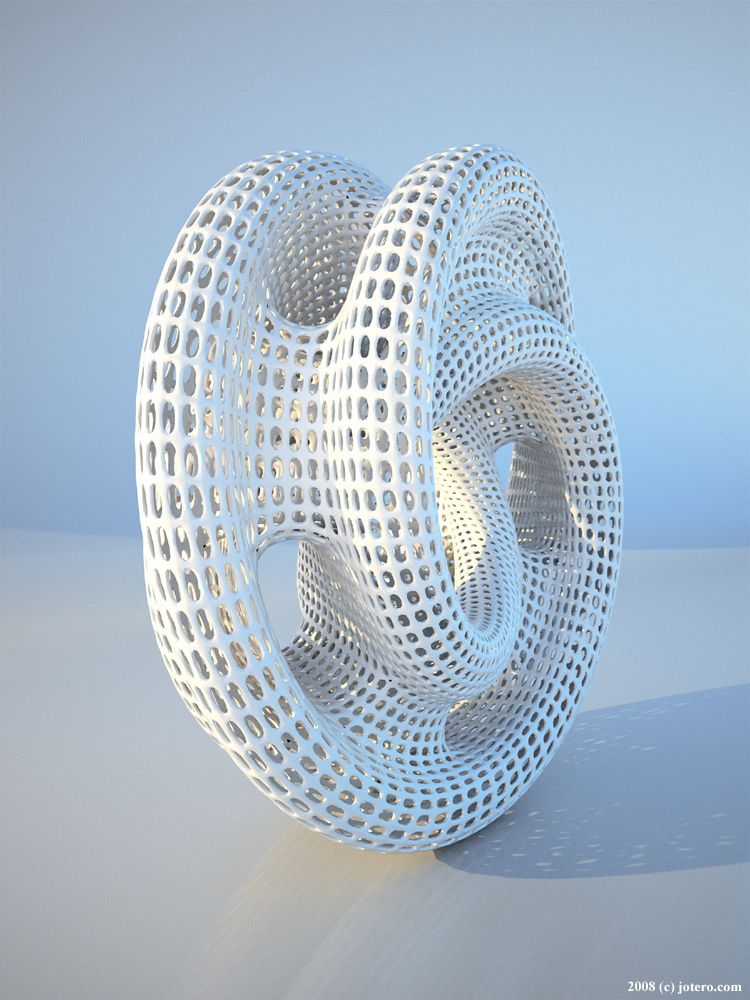
“Bunny” Bunny, by Henry Segerman and Craig Kaplan. The pattern on the bunny consists of copies of the word “bunny.” Listen as the artist describes the sculpture in this YouTube video. Henry Segerman and Craig KaplanTo say that Henry Segerman is schooled in mathematics is an understatement. The 33-year-old research fellow at the University of Melbourne, in Australia, earned a master’s degree in math at Oxford and then a doctorate in the subject at Stanford. But the mathematician moonlights as an artist. A mathematical artist. Segerman has found a way to illustrate the complexities of three-dimensional geometry and topology—his areas of expertise—in sculptural form.
First things first…three-dimensional geometry and topology?
“It is about three-dimensional stuff, but not necessarily easy to visualize three-dimensional stuff,” says Segerman, when we talk by phone. “Topology is sort of split along low-dimensional stuff, which usually means two, three and four dimensions, and then high-dimensional stuff, which is anything higher. There are fewer pictures in the high-dimensional stuff.”
Since 2009, Segerman has made nearly 100 sculptures that capture, as faithfully as is physically possible, some of these hard-to-grasp lower-dimensional mathematical concepts.He uses a 3D modeling software called Rhinoceros, typically used to design buildings, ships, cars and jewelry, to construct shapes, such as Möbius strips, Klein bottles, fractal curves and helices. Then, Segerman uploads his designs to Shapeways.com, one of a few 3D printing services online. “It is really easy,” he says. “You upload the design to their Web site. You hit the ‘add to cart’ button and a few weeks later it arrives.”
Developing Fractal Curves, by Henry Segerman. The artist explains the sculpture, in the center, in this YouTube video. Henry SegermanBefore 3D printing, Segerman built knots and other shapes in the virtual world, Second Life, by writing little bits of programming.
“What cool things can I make in 3D?” he recalls asking himself. “I had never played around with a 3D program before.” But, after a few years, he reached the limit of what he could do within that system. If he wanted to show someone a complicated geometric shape, that person needed to download it to his or her computer, which seemed to take ages.
“That is the big advantage of 3D printing. There is an awful lot of data in there, but the real world has excellent bandwidth,” says Segerman. “Give someone a thing, and they see it immediately, with all its complexity. There is no wait time.”
There is also something to holding the shape in your hand. Generally speaking, Segerman designs his sculptures to fit in someone’s palm. Shapeways then prints them in nylon plastic or a costlier steel bronze composite. The artist describes the 3D printing process, for his white plastic pieces:
“The 3D printer lays down a thin layer of plastic dust. Then, it’s heated up so that it is just under the melting point of plastic.
A laser comes along and melts the plastic. The machine lays down another layer of dust and zaps it with a laser. Do that again and again and again. At the end, you get this vat filled with dust, and inside the dust is your solid object.”
While his primary interest is in the mathematical idea driving each sculpture, and in conveying that idea in as simple and clean a way as possible (“I tend towards a minimalist aesthetic,” he says), Segerman admits that the shape has to look good. A Hilbert curve, the 3-sphere—these are esoteric mathematical concepts. But, Segerman says, “You don’t need to understand all of the complicated stuff in order to appreciate the object.”
If viewers find a sculpture visually appealing, then Segerman has something to work with. “You’ve got them,” he says, “and you can start telling them about the mathematics behind it.”
Here are a few selections from Segerman’s large body of work:
Sphere Autologlyph, by Henry Segerman.
Watch this YouTube video of the artist describing this piece. Henry Segerman Segerman made up the word “autologlyph” to describe sculptures, such as “Bunny” Bunny, pictured at the very top, and this sphere, above. By the artist’s definition, an autologlyph “a word, which is written in a way that is described by the word itself.” With “Bunny” Bunny, Segerman used the word “bunny,” repeated many times over, to form a sculpture of the Stanford Bunny, a standard test model for 3D computer graphics. Then, in the case of this sphere autologlyph, block letters spelling the word “sphere” create the sphere. Minus the bunny, many of Segerman’s autologlyphs have a mathematical slant, in that he tends to use words that describe a shape or some sort of geometric feature.
Hilbert Curve, by Henry Segerman. Watch this video explainer. Henry SegermanThis cube, shown above, is Segerman’s take on a Hilbert curve, a space-filling curve named for David Hilbert, the German mathematician who first wrote about the shape in 1891.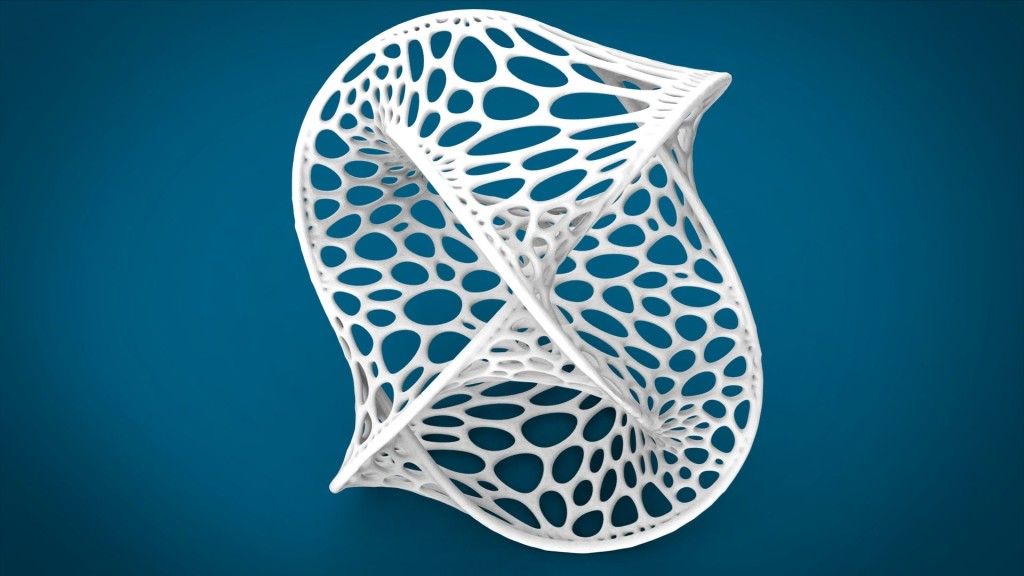
“You start with a curve, really a straight line that turns right angle corners,” says the artist. “Then, you change the curve, and you make it squigglier.” Remember: Segerman does these manipulations in a modeling software program. “You do this infinitely many times and what you get at the end is still some sense a one dimensional object. You can trace along it from one end to the other,” he says. “But, in another sense, it looks like a three-dimensional object, because it hits every point in a cube. What does dimension mean anymore?” Hilbert and other mathematicians became interested in curves like these in the late 19th century, since the geometries called into question their assumptions about dimensions.
“I had been looking at this thing on a computer screen for a year, and when I first got it from Shapeways, and picked it up, it was only then that I realized it was flexible. It is really springy,” says Segerman. “Sometimes the physical object surprises you. It has properties that you didn’t imagine.
”
Round Klein Bottle is a sculpture, much larger than Segerman’s typical pieces, that hangs in the Department of Mathematics and Statistics at the University of Melbourne. (The artist applied a red spray dye to the nylon plastic material for effect.) The object itself was designed in something called the 3-sphere. Segerman explains:
“The usual sphere that you think of, the surface of the earth, is what I would call the 2-sphere. There are two directions you can move. You can move north-south or east-west. The 2-sphere is the unit sphere in three-dimensional space. The 3-sphere is the unit sphere in four-dimensional space.”
In the 3-sphere, all the squares in the grid patterning of this Klein bottle are equal in size. Yet, when Segerman translates this data from the 3-sphere to our ordinary three-dimensional space (Euclidean space) things get distorted.
“The standard Mercator map has Greenland being huge. Greenland is the same size as Africa , whereas in reality, Greenland is much smaller than Africa. You are taking a sphere and trying to lay it flat. You have to stretch things. That is why you can’t have a map of the world which is accurate, unless you have a globe,” says Segerman. “It is exactly the same thing here.”
Segerman is now toying with the idea of moving sculptures. Triple Gear, shown here, consists of three rings, each with gear teeth. The way it is set up, no single ring can turn on its own; all three have to be moving simultaneously. As far as Segerman knows, no one has done this before.
“It is a physical mechanism that would have been very difficult to make before 3D printing,” says the artist. “Even if someone had the idea that this was possible, it would have been a nightmare to try to build such a thing.
”
Recommended Videos
3d Math - Etsy.de
Etsy is no longer supporting older versions of your web browser in order to ensure that user data remains secure. Please update to the latest version.
Take full advantage of our site features by enabling JavaScript.
Find something memorable, join a community doing good.
( 746 relevant results, with Ads Sellers looking to grow their business and reach more interested buyers can use Etsy’s advertising platform to promote their items. You’ll see ad results based on factors like relevancy, and the amount sellers pay per click. Learn more. )
Mathematics in 3D: Mathematical Sculptures by Henry Segerman
The artist turns abstract mathematical concepts into real and fascinating physical objects.
Roman Fishman
According to legend, Pythagoras was the first to discover that two equally stretched strings make a pleasant sound if their lengths are related as small integers. Since then, people have been fascinated by the mysterious connection between beauty and mathematics, the completely material harmony of forms, vibrations, symmetry - and the perfect abstraction of numbers and relationships. This connection is ephemeral, but tangible, and it is not for nothing that artists have been using the laws of geometry for many years and are inspired by mathematical laws. It was difficult for Henry Segerman to turn down 3D mathematics as a source of ideas: after all, he is a mathematician both by vocation and by profession.
Fractals
“I was born into a family of scientists, and I think that my interest in everything that requires advanced spatial thinking is connected precisely with this,” says Henry: this is how he explains his later passion for mathematical sculptures.
Today he is already a graduate of the master's program at Oxford and doctoral studies at Stanford universities, and holds the position of associate professor at the University of Oklahoma. But a successful scientific career is only one side of his multifaceted personality: more than 12 years ago, the mathematician began to arrange artistic actions ... in the virtual world of Second Life. This 3D simulator with social network elements was very popular at that time, allowing users not only to communicate with each other, but also to equip their virtual “avatars” and zones for entertainment, work, etc.
Name: Henry Segerman
Year of Birth: 1979
Education: Stanford University
City: Stillwater, USA
Creed: "Take just one idea, but show it as clearly as possible"
Segerman came with geometry in 3D, armed with formulas and numbers, and arranged his virtual world in a mathematical way, filling it with unprecedented fractal figures, spirals and even tesseracts, four-dimensional hypercubes.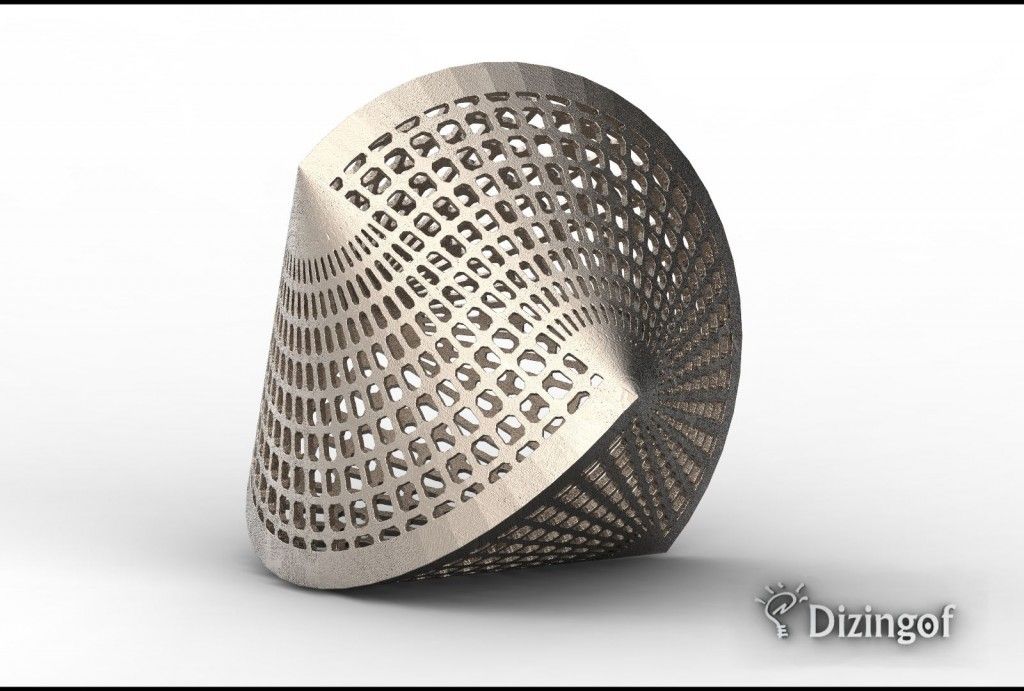
two-dimensional, flat screen,” notes the artist.
However, he liked working with material sculptures much more. “A huge amount of information is constantly circulating around us,” says Segerman. “Fortunately, the real world has a very large bandwidth, which is still unattainable on the Web. Give a person a finished thing, an integral form - and he will perceive it immediately in all its complexity, without waiting for loading. So since 2009, Segerman has created a little over a hundred mathematical sculptures, and each of them is a visual and, as far as possible, an accurate physical embodiment of abstract mathematical concepts and laws.
Polyhedra
The evolution of Segerman's artistic experiments with 3D printing mimics the evolution of mathematical ideas in a strange way. Among his first experiments were the classical Platonic solids, a set of five symmetrical figures composed of regular triangles, pentagons and squares.
Behind them, among his mathematical sculptures, were semi-regular polyhedra - 13 Archimedean solids, the faces of which are formed by unequal regular polygons.
Already these simple geometric shapes in 3D graphics, having migrated from two-dimensional illustrations and the ideal world of imagination to three-dimensional reality, arouse inner admiration for their laconic and perfect beauty. “The connection between mathematical beauty and the beauty of visual or sound works of art seems to me very shaky. After all, many people are acutely aware of one form of this beauty, completely unaware of the other. Mathematical ideas can be translated into visible or sound forms, but not all, and not as easy as it might seem, ”adds Segerman.
Classical geometry in 3D graphics was soon followed by more and more complex forms, up to those that Archimedes or Pythagoras could hardly have thought of - regular polyhedra that fill the Lobachevsky hyperbolic space without a gap.
Such figures with incredible names like “order 6 tetrahedral honeycombs” or “hexagonal mosaic honeycombs” cannot be imagined without having a visual picture at hand. Or one of Segerman's sculptures that represent them in the three-dimensional Euclidean space we are used to.
The artist's work begins with a 3D mathematical model, which he builds in the Rhinoceros professional package. By and large, this is where it ends: the actual production of sculptures, printing the model on a 3D printer, Henry simply orders through Shapeways, a large online community of 3D printing enthusiasts, and receives a finished object made of plastic or a metal matrix composite based on steel and bronze. “It's very easy,” he says. “You just upload the model to the site, click the "Add to Cart" button, place an order - and in a couple of weeks it will be delivered to you by mail."
Beauty
Ultimately, the evolution of Segermann's mathematical sculptures leads us into the complex and fascinating realm of topology.
This branch of mathematics studies the properties and deformations of flat surfaces and spaces of different dimensions, and their broader characteristics are important for it than for classical geometry. A cube here can be easily turned into a ball, like plasticine, and a cup with a handle can be rolled into a bagel without violating anything important in them - a well-known example that was embodied in Segerman's elegant "Topological Joke".
“In mathematics, for 3D graphics, an aesthetic sense is very important, mathematicians love “beautiful” theorems,” the artist argues. - It is difficult to determine what exactly this beauty consists of, as, indeed, in other cases. But I would say that the beauty of the theorem is in its simplicity, which allows you to understand something, to see some simple connections that previously seemed incredibly complex. Mathematical beauty can be based on pure, efficient minimalism—and a surprised exclamation: “Aha!”. The profound beauty of mathematics can be frightening, like the icy eternity of the Snow Queen’s palace.
However, all this cold harmony invariably reflects the internal order and regularity of the Universe in which we live. Mathematics is only a language that unmistakably corresponds to this elegant and complex world. Paradoxically, it contains physical correspondences and applications for almost any statement in the language of mathematical formulas and relations. Even the most abstract and "artificial" constructions sooner or later find application in the real world.
Euclidean geometry became a reflection of the classical stationary world, differential calculus was useful to Newtonian physics. The incredible Riemannian metric turned out to be necessary to describe Einstein's unstable universe, and higher-dimensional hyperbolic spaces found use in string theory. In this strange correspondence of abstract calculations and numbers to the foundations of our reality, perhaps lies the secret of the beauty that we necessarily feel behind all the cold calculations of mathematicians, and it is precisely such mathematics that is reflected in 3D graphics.
Math equations can be seen using 3D printing
Archive
they are beautiful. Recently, the Massachusetts Institute of Technology demonstrated several such models. All of them were created by the mathematician Felix Klein and his assistants. Complex shapes, driven by equally complex equations, turned into a series of 3D printed sculptures. By the way, these models were created by hand a hundred years ago.
First, Klein and his team drew the horizontal sections of the planar form of the equations. Then they printed them out of gypsum powder and glued them together. The result is a three-dimensional model of the equation. The finished products were sanded and streaked with black lines to show the essence of each equation.
For example, one of the sculptures in the Institute's collection illustrates the famous Clebsch cubic diagonal surface. Interestingly, its second name is the icosahedral cubic Klein surface. It got its name in honor of the same Klein who created these models, for his contribution to the understanding of surface features.
This sculpture is a cubic algebraic surface, on which 27 complex lines are applied. In addition, 3 out of 27 lines intersect at 10 points, and this is the only surface on which such a phenomenon can be observed.
This is more than an abstract exercise. Creating three-dimensional models of mathematical concepts makes it possible to study them from a completely new perspective, as well as make them more accessible at various levels. If a student of the Faculty of Mathematics can pick up the solution of an equation, then he will better understand how to solve it. He will have new questions. And it doesn’t matter what it will be: a cube for a freshman or a Clebsh diagonal for a graduate. These models, as well as others, are excellent illustrations of the relationship between 2D instructions and 3D ideas.
These sculptures would not be so easy to create if not for 3D modeling and 3D printing technology. Klein cannot be called a slacker. All these sculptures he made personally or with the help of assistants.
His plaster models are in the collections of the Massachusetts Institute of Technology, the University of Arizona, Harvard, and the University of Illinois at Urbana-Champaign.
There are still a few mathematical ideas left in the world that still resist the magic of 3D printing. They will be the next frontier she needs to take. In particular, we are talking about hyperbolic space (a kind of space in which there is the possibility of countless parallel lines drawn on the surface). At the moment, its form has been transferred only with the help of a hook. The idea of this non-Euclidean surface once seemed impossible, but creating it in 3D with a crochet hook (which is another additive manufacturing technique, albeit considered a common knitting tool) has proven that it has a right to exist.
Exploring the possibilities of mathematics available through 3D printing can help us better understand complex shapes such as Calabi-Yau space, which may be the key to understanding the number and shape of quantities in space and time in the universe.
Also, researchers are seriously interested in creating a three-dimensional model of Lorentzian manifolds (see above the computer model and the crocheted version).
The ability of 3D modeling and 3D printing to make mathematical formulas simpler and more understandable by turning them into physical forms holds great potential for learning and further exploration. They may not allow us to make new discoveries, but they will present things familiar to us in a new light, and it will be easier for us to understand them.
Article prepared for 3DToday.ru
Subscribe to the author
Subscribe
Don't want
Even more interesting articles
four
Subscribe to the author
Subscribe
Don't want
If only yesterday 3D printing was the lot of boys of different age groups trying to adapt new.
